Mathematics and applications
An exceptional education and research environment
- 27 different Master 1 (first year) and Master 2 (second year) covering the entire spectrum of fundamental and applied mathematics
- 750 Master students per year
- 50% of Master's students on average choose to pursue a PhD
- Excellent professional integration
- An area of excellence with 13 Fields medals, 3 Abel prizes, and 4 Wolf prizes
- The Université Paris-Saclay covers a large geographical area in the south of Paris (Orsay, Gif-sur-Yvette, Palaiseau, Bures-sur-Yvette, Versailles, Evry) and includes 13 establishments to which some 15 scientific research laboratories are affiliated, supported by research organizations such as the CNRS, INRIA, INRAE and the CEA.
The international recognition of the mathematical community of the Université Paris-Saclay makes this place one of the best destinations in the world for young talents in search of a high-level mathematical education.
The largest Master's program in Mathematics in the world
The "Mathematics and Applications" master's degree is the largest Master's program in the world, thanks to the joint offer of the Université Paris-Saclay and the Institut Polytechnique de Paris.
The diversity of the M1 (first year) and M2 (second year) is based on the specificities of the 15 laboratories and covers the entire spectrum of fundamental and applied mathematics. Some M1 and M2 take place on several campuses, with different curricula and teachers.
In some cases, there is a first and second year associated:
- M1 + M2 Algèbre Appliquée
- M1 + M2 Analyse, Modélisation, Simulation
- M1 + M2 Formation à l'enseignement supérieur en Mathématique
- M1 + M2 Ingénierie Statistique, Actuariat, Data Science
- M1 + M2 Mathématiques de l'Intelligence Artificielle
- M1 + M2 Mathématiques et Apprentissage Statistique
Graduates in this discipline have an excellent mathematical background and real experience in research, both academic and industrial.
The majority of graduates teach and develop their research in the best academic institutions in the world. Another part leads a brilliant career in the private sector, in particular in the technology industry.
The first year (M1) is designed to help the students steer their studies in a chosen direction; however, all first years (M1s) can potentially lead to all second years (M2s) because applications are examined on a case-by-case basis.
In some cases, the courses are laid out over two years: doing an M1 associated with an M2 (of the same name) secures the passage in the associated M2, but it is also quite possible to enter this M2 via another M1.
For more details about the content of the courses, please consult the tab "Year 1 & 2 Master's Programmes".
This Master's degree is a real springboard for pursuing an academic or corporate doctorate. The training programs are very strongly research-oriented and include a wide variety of advanced courses, as well as research seminars, supervised projects, and internships in laboratories or companies. Much time is devoted to personal research.
It is during the second year (M2) that the student chooses a subject and a thesis director. Some students have a mentor, who plays a crucial role in guiding them and helping them make choices.
- Institut Polytechnique de Paris
- For an entry in the first year (M1): undergraduate degree in mathematics or equivalent. For more details, consult the web page of each course in the tab "Year 1 & 2 Master's Programmes".
- For an entry in the second year (M2): M1 of Mathematics or equivalent. For more details, see the web page of each course in the tab "Year 1 & 2 Master's Programmes".
The quality of student recruitment - supported by the FMJH's international mobility grants - is unequaled. The mix of student populations is one of the remarkable points of this master's program.
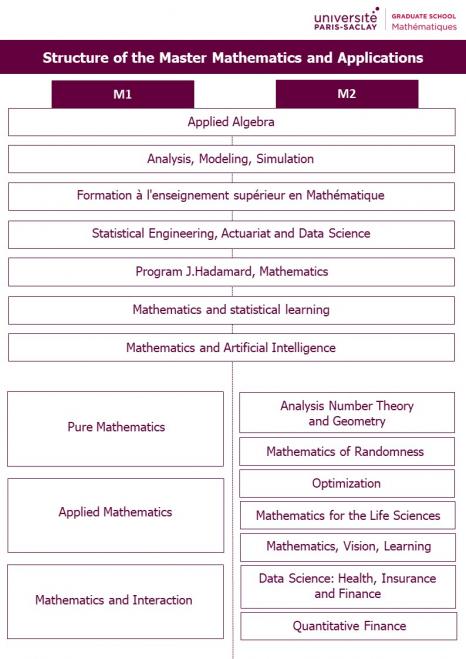
Diagram of the different possible courses within the Mathematics and Applications master's degree.
How to apply?
To apply, please consult the tab "Year 1 & 2 Master's Programmes" and choose the course you are interested in. Before applying, please consult with attention the application procedure.
Scholarships and financial aid
- The FMJH (Fondation Mathématique Jacques Hadamard) Sophie Germain Master's Scholarships for Excellence offer funding for one to two years to French or foreign students.
- Some scholarships are only available to international students: please consult the "international" tab to learn more.
- Other scholarships and funding are offered to French or foreign students by the Université Paris-Saclay: the master's program managers select the students and apply for the master's scholarships on their behalf.
- The Université Paris-Saclay offers other grants (housing allowances, computer purchase...) for master students
IMPORTANT : You must apply for the scholarship and the master in parallel.
Master and use high-level mathematical tools and methods
Design and write a rigorous mathematical procedure
Understand and mathematically model a problem in order to resolve it.
Analyse a research paper with a view to its construct and use
Analyse the data and implement digital simulations
Clearly explain a theory and mathematical results
Financial aids for international students
FMJH (Fondation Mathématique Jacques Hadamard) scholarships for international students
- FMJHCare scholarships: the FMJH offers scholarships to enable excellent foreign students with limited resources to join the Bachelor's or Master's programs in mathematics within its scope.
- The FMJH Sophie Germain Master's Scholarships offer financial aids for one to two years to French or foreign students.
- The FMJH PhD track scholarships allow foreign students with very high potential to obtain financial support for the duration of their studies, from M2 to PhD.
Specific grants for international students at the Université Paris-Saclay
- The Université Paris-Saclay IDEX international scholarships provide support to international students of the highest international level, in particular to those who wish to develop a doctoral project at the end of the master's program.
Specific Campus France scholarships for international students
- The EIFFEL Scholarship Program is developed by the French Ministry of Europe and Foreign Affairs to enable French higher education institutions to attract the best foreign students to their master's and doctoral programs.
Graduate School of Mathematics support and general information for international mathematicians
Need help preparing for your arrival in France? Are you lost in all the steps to take during your stay? No need to panic! We have prepared a summary for you.
Visit the GS web page dedicated to the welcome of international mathematics students to learn more about the E-international welcome office, the Buddy Programme, French courses, the Orsay/Versailles/Evry campuses, the GATE, welcome events...
Université Paris-Saclay also provides all the information you need to start your stay in the best conditions (visa/residence permit, French courses, health restrictions, etc.)
IMPORTANT
You must apply for the scholarship and the master's program at the same time. These are two different but complementary procedures.
Master 1
Master 2
Graduate School of Mathematics
The Master "Mathematics and Applications" is attached to the Graduate School of Mathematics.
The Graduate School of Mathematics federates all research and training activities (Master and post-master) in mathematics. It brings together mathematicians from the Université Paris-Saclay working on a broad spectrum ranging from fundamental to more applied questions, including mathematics at interfaces.
It also maintains and develops relationships with socio-economic partners to ensure a good understanding of the operational issues and scientific questions raised.
The Graduate School is constantly adapting with the skill to the evolution of our world, in particular by evolving its training to meet the new needs of society and new recruitment requirements.